Home » EasyPhys » From the Universe to the Atom » Origins of the Elements » Analyse and apply Einstein’s description of the equivalence of energy and mass and relate this to the nuclear reactions that occur in stars
Analyse and apply Einstein’s description of the equivalence of energy and mass and relate this to the nuclear reactions that occur in stars
Einstein’s equation states that if m amount of mass was completely converted to energy, the total amount of energy released would be E = mc2
- In fusion reactions, the final products have a lighter total mass than the total mass of original products.
- This is called the mass defect , denoted by

- The energy released can be calculated as

- The earliest understanding of what provides energy in the stars came when Cecilia Payne , using spectroscopy discovered that Sun is composed mostly of Hydrogen .
- The extreme heat in the sun due to change of gravitational potential energy to thermal energy, releases the electrons from the atoms and creates a ball of plasma , ionised hydrogen and helium nuclei.
- One possible nuclear reaction could provide energy to the stars was the proton-neutron fusion.

- The deuteron formed has less mass than the original proton and neutron .
- This mass defect is converted to energy, according to the equation
 .
. - The energy released with every fusion is about 2.2 MeV
- But this reaction cannot be the major source of energy as there aren’t enough neutrons in the stars to sustain the reaction.
- Since majority of the element in the stars are Hydrogen atoms, proton-proton fusion is likely the major cause of energy release.
- Although proton-proton electrostatic repulsion is very strong, the extreme conditions in stars help the protons overcome this force and get close enough for strong nuclear force to act.
- As they fuse the to form deuterium, the electrostatic repulsion doesn’t play a part anymore as one of the protons change into a neutron.

- The energy released comes from the difference in mass of the original products from the final products, according to the equation :

- The positron gets annihilated by an electron :

- The deuterium then further fuses with another proton to form Helium
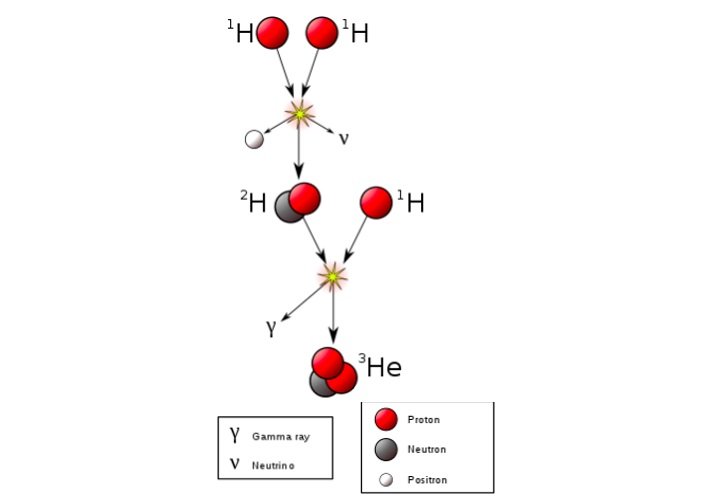
- Depending on the condition of the star (temperature and pressure) , the
 may fuse to form
may fuse to form  through various intermediary steps.
through various intermediary steps.
Extract from Physics Stage 6 Syllabus © 2017 NSW Education Standards Authority (NESA)
